Do EnChromas work on screens? There have been conflicting reports on this, even from EnChroma themselves, where I have seen them claim they would and would not work. Of course, it may well depend on the type of lens in questions, but I always thought it an interesting question. I found a paper today that confirmed my intuition.
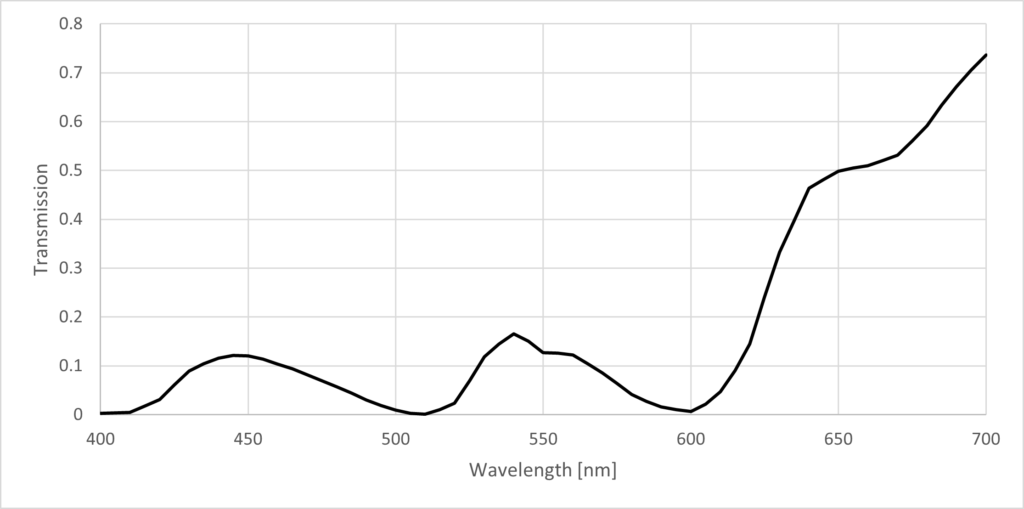
Their graphics were very useful but awful from a design perspective, so I’ve digitized and regraphed them. The study used EnChroma outdoor (Cx3) lenses, that have a transmission that is shown below with notches (absorption) maximally at 600 and 510 nm.
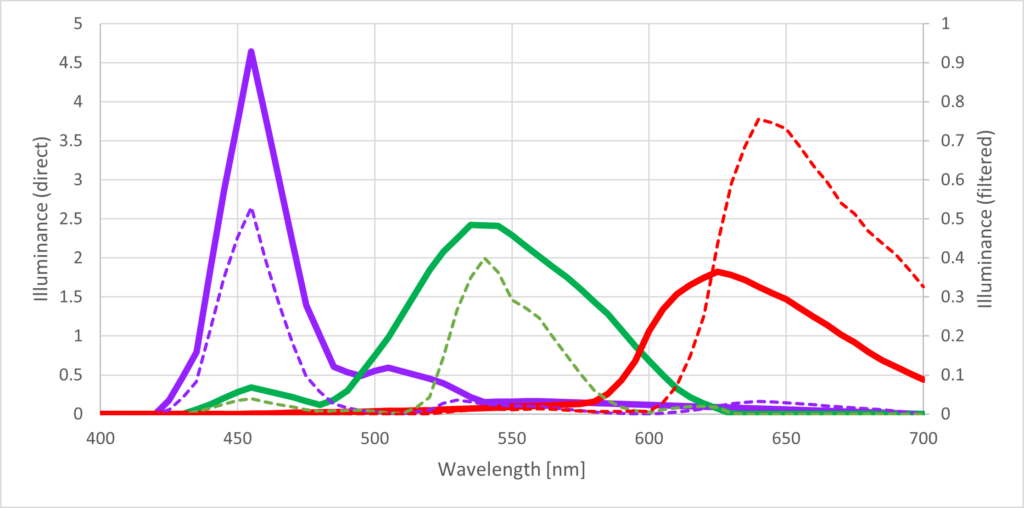
An Acer IPS 34″ display was used in the study. The spectra of each of the 3 primaries was measured and are shown in the solid lines below. The screen is not considered to have a very large color gamut. Recall that the color gamut of a screen is mostly proportional to how narrowband the primaries’ emission spectra are. The broader they are (the more they overlap) the smaller the gamut will be and the less saturated colors can be displayable. The largest gamut would be generated by primaries that are monochromatic, such as a laser projector with primaries at exactly 450, 540 and 650 nm. In that case, the corners of the triangular gamut would all rest on the spectral locus of the chromaticity diagram (and be maximally large).
So how can we improve this Acer screen? Well, we’d definitely want to start by narrowing those emission spectra, and push the vertices of the color gamut closer to the spectral locus, thereby increasing the gamut size. Enter EnChroma glasses. Viewing the screen with EnChromas on is the same thing as putting a film of EnChroma material over the screen, and in that case, the effective, filtered primary emission spectra are equal to the product of the direct spectra and the lens transmission. These filtered primaries are represented by the dashed lines below.
First, notice how the peaks, most notably the green peak of the M-cone, have become much thinner. The red has also become effectively thinner, when you consider that all of the high wavelength emissions get truncated by your eye just not being sensitive to them. The blue (represented by purple because I’m colorblind) doesn’t appear that much thinner. Therefore, we expect to see the red and green vertex to move towards the spectral locus and increase the size of the gamut.
Second, notice that the area under each curve is approximately equal for the direct spectra, but once filtered, the red emissions dominate. This is also clear by looking at the transmission spectrum. It simply means that the EnChroma lenses are red-tinted, at least here. I have usually seen references to the tint as being magenta, but its a bit hard to know which lens version each viral influencer or Reddit skeptic has got their hands on.
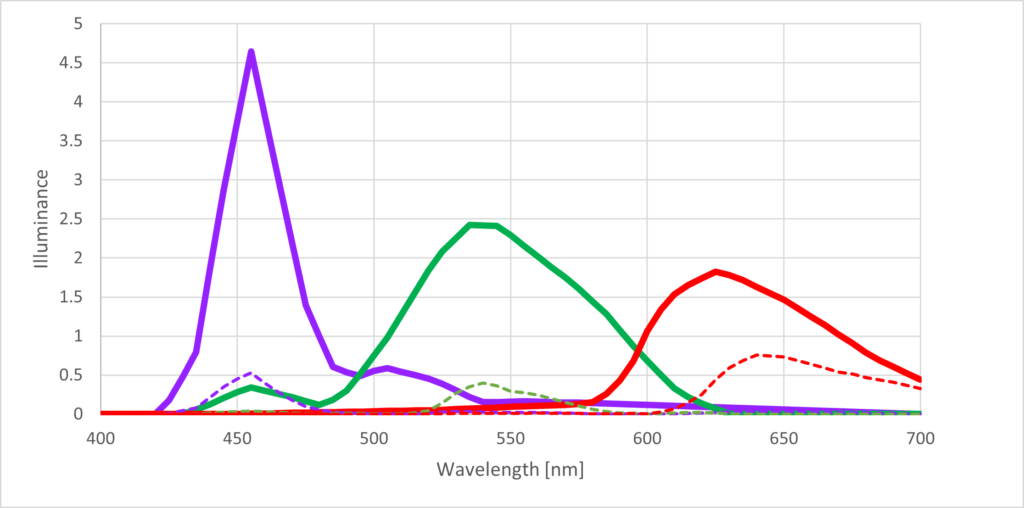
Third, notice that I have the direct and filtered spectra on different y-axis to make the comparison easier. In fact, when we place them on the same y-axis, as below, the emission of each sub-pixel (R, G, B) is much weaker. This is pretty obvious, since the outdoor EnChroma lenses are SUNGLASSES after all, and the transmission spectra shows the transmission to only be about 10% on average, meaning 90% of the light is absorbed or reflected.
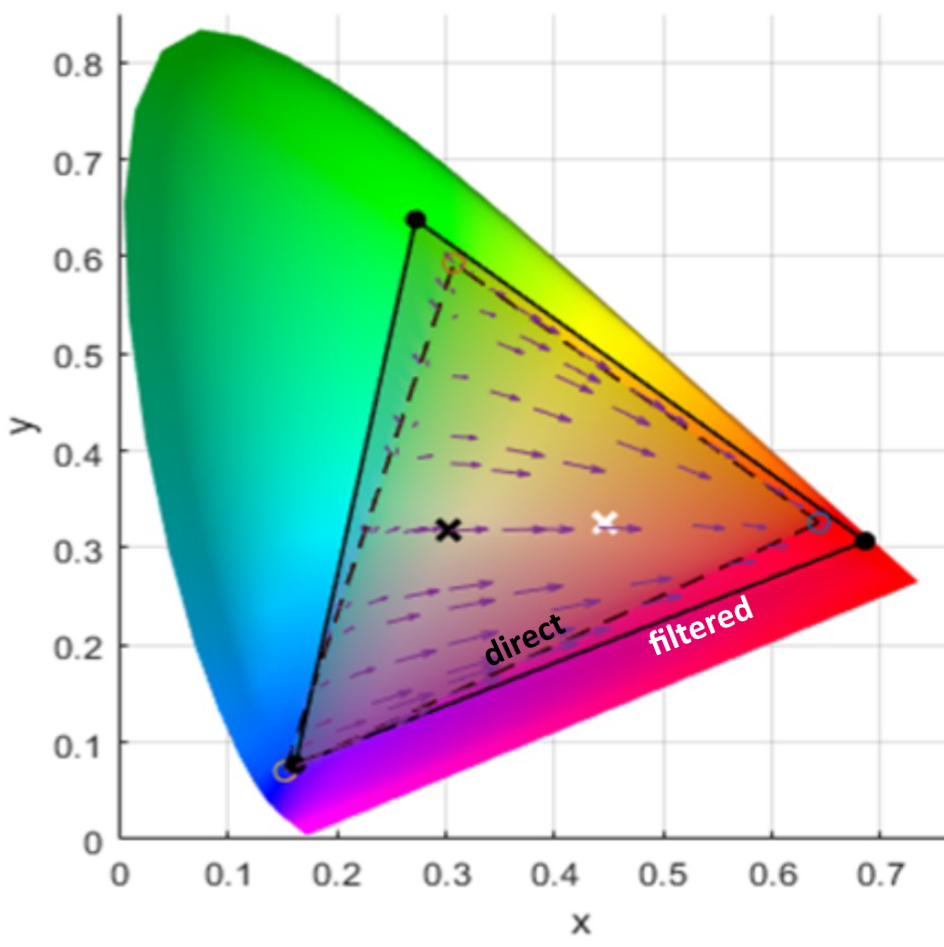
The graph below is what we get when we plot the display’s direct (unfiltered) and filtered gamuts on the chromaticity diagram. The direct gamut is given by the dashed line. The filtered gamut is given by the solid line, and is clearly a little bit larger. The vertices represented by the green and red primaries have moved closer to the spectral locus (the edge of the colored area) and the red has moved to longer wavelengths (towards the right corner), as we expected.
The black x marks the neutral (white) point of the direct gamut, and the white x marks the neutral point of the filtered gamut, which is shifted far towards the red side, indicating the red tint. The arrows within the gamut also show how each color is shifted as a result of the filtering, with pretty much everything being turned redder, with the slight exception of the greenest/cyanest colors that already lack red, so are turned ever so slightly more saturated green/cyan.
This red tint shouldn’t necessarily be a problem though, since your chromatic adaptation should be able to compensate for it after wearing the glasses for a while, slowly shifting the neutral point back to where it was and “subtracting” the red “cast” from your vision. At least that’s the idea.
So is that it? EnChromas increase your gamut when looking at a screen? Not so fast…
First, the paper also applied the EnChromas to a Google Pixel 6, which has an AMOLED “wide gamut” display that already has primaries with narrower emission spectra. Viewing the Pixel display through the EnChromas didn’t actually increase the already large Gamut at all, so it seems the trick only “works” for displays that can only maximally display sRGB (a standard gamut size) or worse.
Pilestones
The paper also put Pilestone glasses over both displays in the same way and showed that while they slightly increased the purple end of the gamut, they severely truncated the green side of the gamut.
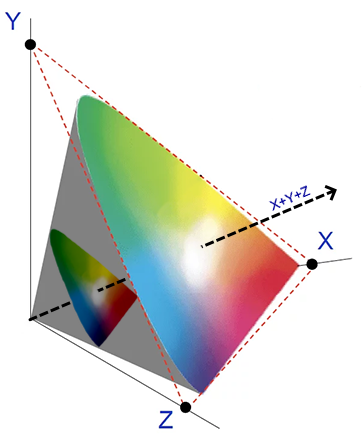
Second, the 2D chromaticity diagram represents chromaticities… duh… which is all colors of the same luminance. If you imagine all of the colors a trichromat can see, at different luminances, we get a 3D color solid, represented below by the gray shape. The brightness/luminance of the colors increases along the vector X+Y+Z, and each value along this vector is associated with a slice of the color solid normal to the vector, each of which is essentially its own chromaticity diagram. However, notice as you get to smaller luminances how the chromaticity slice shrinks, indicating that there are fewer distinct colors that can be distinguished in the entire visual gamut. We all know this intuitively: any color task gets harder when the lights go dim.
On the same chromaticity diagram, above, it seems clear that the filtered gamut is larger, but we also have to remember that we are wearing sunglasses inside. The screen is much darker and therefore the corresponding chromaticity diagram is smaller. You therefore get a bigger slice of a smaller pie, and the number of total number of unique colors you can differentiate with the sunglasses on still goes down.
Just like with chromatic adaptation, your visual system is actually pretty good at compensating for the change in brightness, such as by dilating the pupil. However, this compensation only works to a degree. If you wear your sunglasses outside, where there is already more than enough light, a loss of 90% of the light still leaves more than enough for your eye and there is probably not going to be any change to the size of your visual gamut. Past a certain luminance, you kind of “max out” the size of your visual gamut (and it can even start to decrease again if things are too bright!). However, if you are looking at a display at 10% brightness, that dimming is more than your eyes can compensate and the visual gamut is certainly smaller.
So what about the indoor EnChromas? These don’t filter out nearly as much of the light and are often recommended for screen-work, so why didn’t the paper go with testing indoor glasses? Well, as the filtering of light is the working principle of these notch-filter glasses, a lighter filter just means a weaker color effect. The indoor glasses would have almost no effect on “shaping” the primaries’ emission spectra and while the visual gamut would not shrink much, the effective display gamut would not increase much either.
Want to boost the colors you can see on your display? Buy a “wide gamut” display and/or boost the saturation past sRGB in your settings. It will do way more than EnChroma lenses ever could.
Leave a Reply